CBSE Class 10th Trigonometric Ratios Details & Preparations Downloads

Welcome to the realm of triangles and angles, where the ancient art of trigonometry takes center stage. In this exploration, we delve into the heart of trigonometry, unraveling the secrets of trigonometric ratios. These ratios, rooted in the relationships between sides and angles, form the backbone of this mathematical discipline. Join us on this journey as we demystify trigonometric ratios, unlocking a world of practical applications and mathematical elegance.

Mastering Trigonometric Ratios Your Comprehensive Guide with CBSE NCERT Downloads

Understanding the Basics

At the core of trigonometry lies a set of fundamental ratios: sine, cosine, and tangent. These ratios establish connections between the angles of a triangle and the lengths of its sides. As we embark on this adventure, we'll break down each ratio, exploring its definitions, properties, and real-world significance.

Sine (sin), Cosine (cos), and Tangent (tan)

Sine (sin): Defined as the ratio of the opposite side to the hypotenuse in a right-angled triangle, sinθ = opposite/hypotenuse.

Cosine (cos): Representing the ratio of the adjacent side to the hypotenuse, cosθ = adjacent/hypotenuse.

Tangent (tan): Tangent is the ratio of the opposite side to the adjacent side, tanθ = opposite/adjacent.

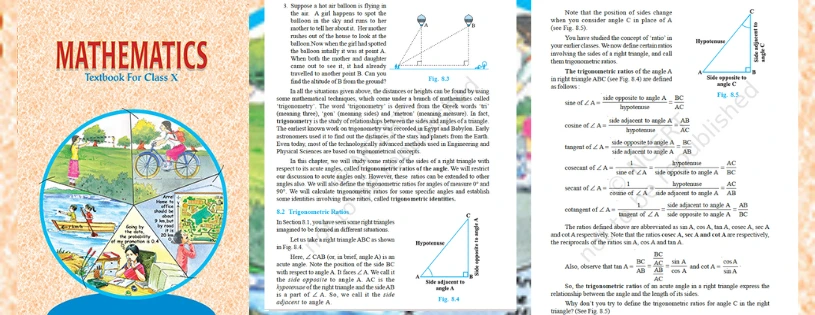
Trigonometric Ratios are defined as the values of all the trigonometric functions based on the value of the ratio of sides in a right-angled triangle. The ratios of sides of a right-angled triangle with respect to any of its acute angles are known as the trigonometric ratios of that particular angle.

The three sides of the right triangle are

• Hypotenuse (the longest side)
• Perpendicular (opposite side to the angle)
• Base (Adjacent side to the angle)

How to Find Trigonometric Ratios?

Consider a right-angled triangle, right-angled at B.

With respect to ∠C, the ratios of trigonometry are given as:

• sine: The sine of an angle is defined as the ratio of the side opposite(perpendicular side) to that angle to the hypotenuse.
• cosine: Cosine of an angle is defined as the ratio of the side adjacent to that angle to the hypotenuse.
• tangent: The Tangent of an angle is defined as the ratio of the side opposite to that angle to the side adjacent to that angle.
• cosecant: Cosecant is a multiplicative inverse of sine.
• secant: Secant is a multiplicative inverse of cosine.
• cotangent: Cotangent is the multiplicative inverse of the tangent.

Download Mathematics notes

The above ratios are abbreviated as sin, cos, tan, cosec, sec, and tan respectively in the order they are described. So, for Δ ABC, the ratios are defined as:

sin C = (Side opposite to ∠C)/(Hypotenuse) = AB/AC

cos C = (Side adjacent to ∠C)/(Hypotenuse) = BC/AC

tan C = (Side opposite to ∠C)/(Side adjacent to ∠C) = AB/BC = sin ∠C/cos ∠C

cosec C= 1/sin C = (Hypotenuse)/ (Side Opposite to ∠C) = AC/AB

sec C = 1/cos C = (Hypotenuse)/ (Side Opposite to ∠C) = AC/BC

cot C = 1/tan C = (Side adjacent to ∠C)/(Side opposite to ∠C)= BC/AB

In right Δ ABC, if ∠A and ∠C are assumed as 30° and 60°, then there can be infinite right triangles with those specifications but all the ratios written above for ∠C in all of those triangles will be same. So, all the ratios for any of the acute angles (either ∠A or ∠C) will be the same for every right triangle. This means that the ratios are independent of the lengths of the sides of the triangle.

Trigonometry Applications

Trigonometry is one of the most important branches of mathematics. Some of the applications of trigonometry are:

• Measuring the heights of towers or big mountains

• Determining the distance of the shore from the sea

• Finding the distance between two celestial bodies

• Determining the power output of solar cell panels at different inclinations

• Representing different physical quantities such as mechanical waves, electromagnetic waves, etc.

It is evident from the above examples that trigonometry has its involvement in a major part of our day-to-day life and much more. In most of the applications listed above, something was being measured and that is what trigonometry is all about.

CBSE Class 10th Downloadable Resources: 

Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going. 

Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain. 

SAMPLE PRACTICE QUESTION

Q1: Prove the trigonometric identity: \( \sin(A + B) = \sin A \cos B + \cos A \sin B \).?

Ans: To prove \( \sin(A + B) = \sin A \cos B + \cos A \sin B \), we use the sum-to-product formula for sine.

Q2: Simplify the expression: \( \frac{\tan\theta}{1-\cot\theta} + \frac{\cot\theta}{1-\tan\theta} \).?

Ans: Combine fractions and simplify using trigonometric identities to get the final expression in terms of sine and cosine.

Q3: Show that \( \csc\theta - \sin\theta \cot\theta = \cos\theta \).?

Ans: Start by expressing everything in terms of sine and cosine, then simplify and manipulate using trigonometric identities.

Q4: Derive the double angle identity for cosine: \( \cos(2\theta) = \cos^2\theta - \sin^2\theta \).?

Ans: Utilize the double angle formula for sine and cosine and manipulate the expression to prove the given identity.

Q5: Find the general solution for \( \cos^2\theta - \sin^2\theta = \frac{1}{2} \).?

Ans: Solve the trigonometric equation for all possible values of theta, considering the periodic nature of trigonometric functions.

Download Question Bank