CBSE Class 10th Trigonometric Ratios of Some Specific Angles Details & Preparations Downloads
Trigonometric ratios of some specific angles: In Mathematics, trigonometry is a branch that deals with the sides and angles of a right triangle. The three basic trigonometric functions are sine (sin), cosine (cos), and tangent (tan). The other trigonometric functions that are derived from the basic trigonometric functions are cosecant (cosec), secant (sec), and cotangent (cot). In this article, we will learn how to derive the trigonometric ratios of some specific angles with a complete explanation.
Mastering Trigonometric Ratios of Specific Angles Dive into Precision with CBSE NCERT Downloads
Trigonometric Ratios of Some Specific Angles – Definition
Trigonometric ratios of some specific angle are defined as the ratio of the sides of a right-angle triangle with respect to any of its acute angles. Trigonometric ratios of some specific angles include 0°, 30°, 45°, 60° and 90°. Now, let us learn how to find the trigonometric ratios of these angles in detail.
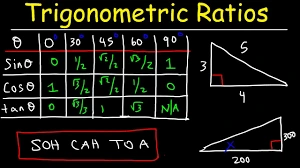
Trigonometric Ratios of 45°
Consider a right-angle triangle ABC as shown in the figure.
Here, a triangle ABC is right-angled at B. (i.e) ∠B = 90°.
If one of the angles is 45°, then the remaining angle should be 45°, as the sum of the interior angles of a triangle is 180°.
Therefore, ∠A = ∠C = 45°.
Also, AB = BC.
Now, assume that AB = BC = a
By using Pythagoras theorem, we can say that AC2 = AB2 + BC2
AC2 = a2 + a2
AC2 = 2a2
Therefore, AC = a√2.
Therefore,
sin 45° = BC/AC = a/ a√2 = 1/√2.
cos 45° = AB/AC = a/ a√2 = 1/√2.
tan 45° = BC/AB = a/a = 1
As, cosecant, secant, and cotangent are the reciprocal of sine, cosine, and tangent, respectively, then we can write:
cosec 45° = 1/ sin 45° = √2
sec 45° = 1/ cos 45° = √2
Cot 45° = 1/ tan 45° = 1.
1. The Foundation: Understanding Trigonometric Ratios
Before delving into specific angles, let's establish a strong foundation in trigonometric ratios. We'll revisit the basics of sine, cosine, and tangent, laying the groundwork for our exploration into the intricacies of specific angles.
2. 30°, 45°, 60°: Unraveling Common Angles
Explore the trigonometric ratios associated with special angles – 30°, 45°, and 60°. Discover their significance, applications in real-world scenarios, and how these ratios become invaluable tools in problem-solving and geometric analysis.
3. The Unit Circle: A Visual Guide to Ratios
Enter the world of the unit circle, a powerful tool for visualizing trigonometric ratios. We'll explore how this circle aids in understanding the relationships between angles and ratios, providing a geometric perspective that enhances comprehension.
4. Beyond the Basics: Ratios of Complementary Angles
Delve into advanced concepts as we explore the relationships between trigonometric ratios of complementary angles. Uncover the interconnected nature of these ratios and how they contribute to a deeper understanding of trigonometry.
5. Practical Applications: Bringing Trigonometric Ratios to Life
Witness the real-world applications of trigonometric ratios of specific angles. From engineering and physics to architecture and navigation, we'll showcase how these ratios play a vital role in solving complex problems and making sense of the physical world.
Trigonometric Ratios of Some Specific Angles Examples
Example 1
Determine the value of A and B, if sin (A-B) = ½, cos(A+B) = ½, and 0° < A + B ≤ 90°, where A>B.
Solution
Given that: sin (A-B) = ½
cos(A+B) = ½, and 0° < A + B ≤ 90°, where A>B.
sin (A- B) = ½
Therefore, A-B = Sin-1(½) = 30°….(1)
cos (A+B) = ½
A+B = cos-1(½)
A+B = 60°…(2)
On solving the equation (1) and (2), we get
A = 45° and B = 15°.
Therefore, the values of A and B are 45° and 15°, respectively.
CBSE Class 10th Downloadable Resources:
| 1. CBSE Class 10th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 10th NCERT Books | View Page / Download |
| 3. CBSE Class 10th NCERT Solutions | View Page / Download |
| 4. CBSE Class 10th Exemplar | View Page / Download |
| 5. CBSE Class 10th Previous Year Papers | View Page / Download |
| 6. CBSE Class 10th Sample Papers | View Page / Download |
| 7. CBSE Class 10th Question Bank | View Page / Download |
| 8. CBSE Class 10th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 10th Last Minutes Preparation Resources (LMP) | View Page / Download |
| 10. CBSE Class 10th Best Reference Books | View Page / Download |
| 11. CBSE Class 10th Formula Booklet | View Page / Download |
Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTION
Q1: What are the specific angles commonly used in trigonometry?
Ans: In trigonometry, specific angles include 0°, 30°, 45°, 60°, and 90°, which are fundamental in understanding trigonometric ratios.
Q2: What are the sine, cosine, and tangent ratios for a 0° angle?
Ans: For a 0° angle, the sine (\(\sin\)) is 0, the cosine (\(\cos\)) is 1, and the tangent (\(\tan\)) is 0.
Q3: What are the trigonometric ratios for a 30° angle?
Ans: For a 30° angle, \(\sin(30°) = \frac{1}{2}\), \(\cos(30°) = \frac{\sqrt{3}}{2}\), and \(\tan(30°) = \frac{1}{\sqrt{3}}\).
Q4: What are the trigonometric ratios for a 45° angle?
A: For a 45° angle, \(\sin(45°) = \frac{\sqrt{2}}{2}\), \(\cos(45°) = \frac{\sqrt{2}}{2}\), and \(\tan(45°) = 1\).
Q5: How about the trigonometric ratios for a 60° angle?
Ans: For a 60° angle, \(\sin(60°) = \frac{\sqrt{3}}{2}\), \(\cos(60°) = \frac{1}{2}\), and \(\tan(60°) = \sqrt{3}\).